To get ready for the New Horizons flyby of Pluto (this July!), I’m writing a series on the science of Pluto and the Kuiper Belt.  Here is the next installment.

There are some very strong hints that the planets haven’t stayed in their original orbits and the giant planets tossed around the smaller ones.  These hints will help us understand several cool things about the structure of the Kuiper Belt including how Pluto got into an orbit that is so tilted and resonant with Neptune.  (Orbital resonance was described in a prior post, at this link.)

A Little Orbital Mechanics

Orbital mechanics is the strange physics of how bodies orbit one another. Let’s say you are in a spaceship in low Earth orbit trailing the Space Station, and let’s say you are trying to catch up to it.  You might think you need to go faster, right? Actually you need to slow down. Attempting to slow down makes you you swing closer to the Earth so your potential energy is reduced causing you to actually go faster, and also your orbital path is shorter. You then orbit the Earth in less time than the Space Station, so you come out ahead.  Attempting to speed up makes you go slower and attempting to slow down makes you go faster. Strange, right?

Here’s another weird thing about orbital mechanics:  the combination of real and apparent forces can make you orbit an empty location in space. Centrifugal force is an apparent force you feel when you are spinning.  If you spin a bucket of water over your head, the water feels the centrifugal force holding it in the bucket when it is upside down so it doesn’t fall out onto your head.  When your spaceship is in a circular orbit around the Earth, the centrifugal force the spaceship feels just exactly balances the gravity from the Earth, so it never moves closer to nor farther from the Earth. That balance gives it a circular orbit.  Another apparent force is the Coriolis force.  In the Wizard of Oz, when Dorothy’s house was spinning in the twister, if she tried to walk across the room she would have been thrown toward the wall.  We call that tendency to swerve off a straight line when you’re in a spinning reference frame the Coriolis force.  If you are orbiting the Earth then you will feel the gravity of both the Earth and the Moon, and these two real forces plus the apparent forces will balance each other in five special locations.  These are called the Lagrange points. If you are very close to a Lagrange point but not exactly right at it, then the real and apparent forces will tend to push you back toward the Lagrange point, and they can even make you go into orbit around the Lagrange point. Two of the five Lagrange points are stable so you really will orbit them. The other three are almost stable so you can go around and around for a long time before you finally break free.  This is very cool, because you are orbiting a point in space where nothing exists.  There is no actual gravity from that point to make you orbit it.

Now all of this was to introduce the next concept.  In a recent post I talked about orbital resonances and how the planets give little kicks to each other. Pluto is in a 2:3 resonance with Neptune, so every time Pluto orbits the sun twice, Neptune orbits the sun exactly three times. Therefore, the kicks from Neptune don’t hit Pluto randomly but in an orderly pattern. It turns out this pattern of kicks is stable like the Lagrange points. If Pluto’s orbit wasn’t exactly in the 2:3 resonance — let’s say it completed slightly more than two orbits for Neptune’s three, then the kicks from Neptune would tend to pull Pluto along faster, and since going faster makes it swing farther from the sun it would actually slow down and take longer to go around, which would move it back toward the exact 2:3 resonance. And if Pluto were completing slightly less than two orbits for Neptune’s three, then the kicks would tend to pull Pluto backward as if to slow it down, but that would make it swing closer to the sun so it actually speeds up and moves forward closer to the exact 2:3 resonance. Unlike the Lagrange points, these resonances are not specific locations in physical space. Instead, they are locations in “parameter space.” When the parameters of the orbit (i.e., the semi-major axis, the eccentricity, and so on) are close enough to what they need to be for resonance, then the forces of orbital mechanics will push the planet through this parameter space closer to that special set of parameters. Cool, right?

That’s enough orbital mechanics. Now, what does this have to do with the origins of the Kuiper Belt?

Clues in the Solar System

The planets formed out of a flat disk of gas and dust like a giant Frisbee spinning around the newly forming sun.  Therefore, they orbited in approximately the same, flat plane; we call it the ecliptic plane.  We can find the ecliptic plane when we look at the planets in the night sky. They will all be in the same great circle that seems to go around the Earth, all following approximately the same narrow path crossing the same constellations year after year. That path is the side view of the ecliptic plane. We see it as a side view because the Earth is in the plane along with the other planets.

When planetary bodies get too close to each other they can scatter out of the plane. We call the resulting tilt of their orbit relative to that plane the inclination of the orbit. Consider two cars going the same direction down a long, straight highway. If the cars collide just slightly off-center, they can careen completely off opposite sides of the road, even though they were going pretty straight down the road to begin with.  The slightly off-center alignment gets exaggerated by the crash. Likewise, the planets were not all exactly in the ecliptic plane, but pretty close. When they swing near each other, the gravitational “crash” exaggerates their slightly off-center alignment, throwing them in opposite directions out of the plane, giving them both larger inclinations. Also, if a heavy truck collides with a tiny car, the car will get scattered farther off the road than the truck will.  Likewise, if a smaller body like Pluto interacts with a heavier one like Neptune, Pluto will get the larger inclination as a result.  By looking at the inclinations of the many bodies of our solar system, we can get a clue as to how much scattering has taken place.

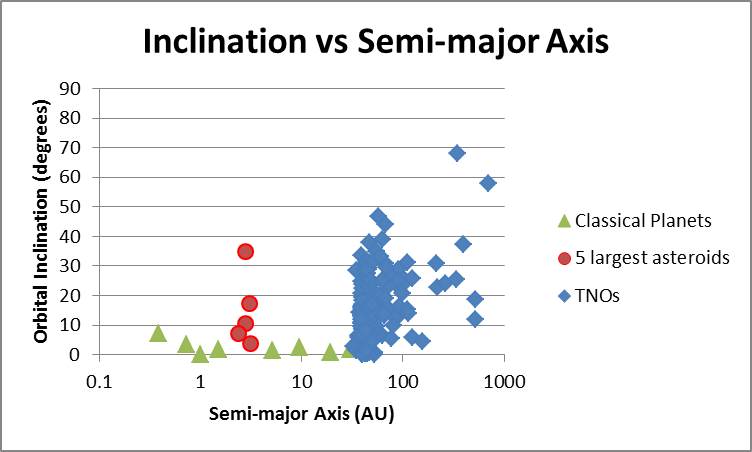
Another clue about scattering in our solar system is the eccentricity of planetary orbits. Eccentricity is defined so that a circular orbit has an eccentricity of zero, while an extremely elliptic orbit approaches the limiting value of one.  Note in the next plot how the TNOs tend to fall on the same arc. That’s because they all have perihelions (closest approaches to the sun) in a narrow range of values near Neptune, which scattered them, but depending on how much they were scattered they can travel varying distances from the sun, and that distance gives them both a higher eccentricity and a higher average distance from the sun (semi-major axis) so the two are mathematically related. Note also the large eccentricity of Mercury, showing how it has been scattered by other bodies.

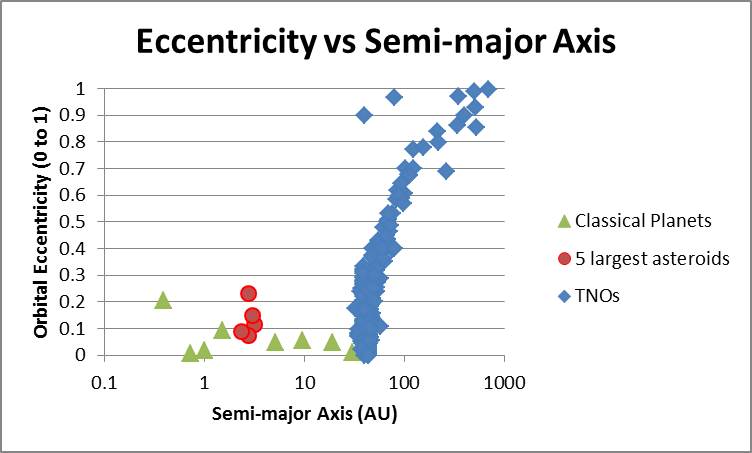
High eccentricity also indicates that bodies have been scattered.

Migration of the Giants

To explain these and many other clues, scientists have come up with models of what apparently happened in the solar system’s past.  Two recent models, the Nice Model and the Nice 2 Model, have been highly successful at explaining many of these things.  (“Nice” is pronounced like “niece” since it refers to the city in France.)

According to these models, several billion years ago the four giant planets were in more circular orbits with smaller inclinations.  Uranus and Neptune were closer to the orbit of Saturn at that time, and the Kuiper Belt was vastly more dense than it is today and much closer to the sun, just outside the orbits of the giants.  Then, Jupiter and Saturn slowly drifted into a 1:2 resonance with each other. That was brought about through the gravitational tugging of the many small bodies around them. Once they reached this resonance, Jupiter and Saturn kicked each other into more eccentric orbits. That made them start kicking Uranus and Neptune, flinging them farther out, plunging them into the heart of the early Kuiper Belt. Then Uranus and Neptune began flinging most of the bodies of the Kuiper Belt far away, out into the Oort Cloud or completely out of the solar system. The survivors — those that were not tossed as violently — are what we see as the modern Kuiper Belt.  Though they weren’t thrown as far, they were still tossed into higher inclinations and higher eccentricities, explaining the shape of modern Kuiper Belt.

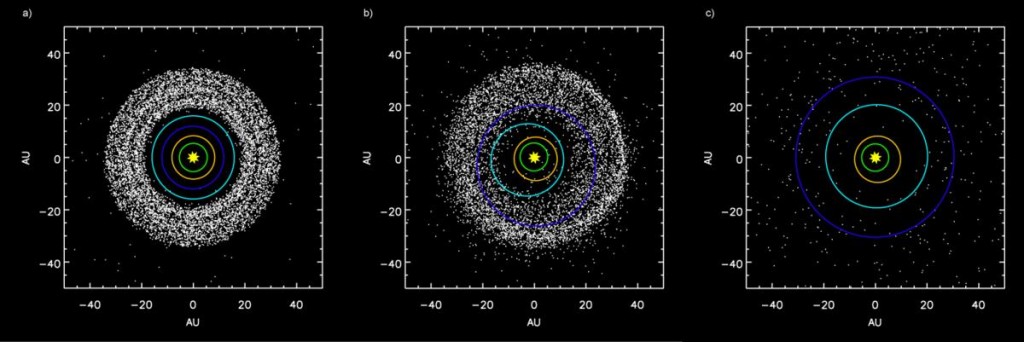
Although I used the word “plunged”, the migration of Neptune and Uranus took tens of millions of years. As they moved, their orbital periods were getting longer. Inevitably, Neptune’s period got close to resonance with one Kuiper Belt body after another.  Some of those bodies were very close to the special location in parameter space for the 2:3 resonance, so the forces of orbital mechanics pushed them even closer to it and they went into orbit around that point. As Neptune kept migrating outwardly, this location in parameter space also moved, but orbital mechanics kept pushing the resonant bodies so they all migrated together. Like a wave moving toward the beach sweeping up the surfers in its path, Neptune swept many bodies into this resonance. This explains why there are so many plutinos:  they didn’t form in that 2:3 resonance; they were swept there one-by-one as Neptune’s influence rolled through the Kuiper Belt like a giant wave.

This 2:3 resonance is not the only one that swept up planetary bodies during the gas giants’ migration. Other resonances are populated as well.  Many of these resonant bodies are likely to be confirmed as dwarf planets when we get a better measurement of their sizes.

Summary

When the New Horizons spacecraft flies past Pluto on its historic encounter, here are some cool things to remember:

1. Pluto likely formed much closer to the sun, along with the other Kuiper Belt Objects. This may help explain why Pluto is mostly rock instead of ice.
2. Pluto (like most Kuiper Belt Objects) has a high inclination and high eccentricity because it was scattered through gravitational encounters with Neptune (and Uranus?) billions of years ago. Its orbit was affected by these encounters more than Neptune’s was affected simply because of their differences in mass.
3. Pluto, and the other bodies in 2:3 resonance with Neptune (the plutinos), were likely swept into this resonance as Neptune migrated away from the sun several billion years ago, and once they got into the resonance they rode the wave until Neptune finished migrating.
4. Many clues about our solar system are in the Kuiper Belt, so the New Horizons mission may be the first of a new wave of planetary spacecraft going out to this third zone of the solar system.

If you enjoyed this post, please share it with others! Do you have questions or comments? Please let me know, below.

Note: this article was amended. Originally it stated that objects farther from the sun have higher velocities yet take longer time to complete an orbit only because the distance is longer. Thanks to reader Robert who pointed out my error. In fact, objects go more slowly the farther they are from the body they are orbiting, their velocities scaling as v=GM/Sqrt(R), where G and M are the gravitational constant and mass of the object they orbit, and R is the semi-major axis, sort of like an average orbital radius. Velocity decreases as R increases. Therefore, as you thrust to try to “speed up”, you are fighting “uphill” against gravity the whole way and you are actually slowing down.  The lost kinetic energy and the new work from your thrusting both go into gravitational potential energy.